【研究意义】亚晶界是由一系列位错按特定的方式排列而成的一种面缺陷,广泛存在于晶粒内部,其结构与迁移方式对材料的力学行为有重要的影响[1]。通常认为亚晶界是一种位错源,即位错在应变作用下发生分离作用,形成新晶体[2],就是所谓的亚晶界,该过程实质上是生成了亚晶粒[3]。在应力作用下,位错发生迁移进而导致亚晶界的湮没。但是在现有实验条件下较难观察到亚晶界上位错的分布以及施加应力后的动态过程,所以我们选用计算机模拟的方法来研究这个问题。现在已经有很多学者用各种计算机模拟方法对小角双晶位错进行相关研究,例如分子动力学(Molecular dynamic simulations,MD)法[4-5],晶体相场(Phase-Field Crystal,PFC)法[6-10]等。但是由于MD方法所施加的应变作用,量级上往往与实际实验情况相差较大[1],因此该方法存在局限性,而Elder等[11]基于密度泛函理论提出的晶体相场模型能够在原子尺度和扩散时间尺度上模拟材料的微观结构转变和演化,具有较大的优势,并且已经有很多人对这个模型进行了应用[12-17],所以本研究也采用晶体相场法。【前人研究进展】杨涛等[1]用晶体相场法研究了应力诱发双位错亚晶界湮没,并得到了不同应力和温度下对于位错淹没情况的影响,以及高英俊等[2]用晶体相场法研究高温应变下晶界湮没机理,用晶粒长大来解释能量变化曲线,使我们对亚晶界的湮没机制有了更深的理解。【本研究切入点】关于小角双晶被施加压力后亚晶界上位错分布及动态过程变化特征还未见报道,本研究将用亚晶界的湮没来解释自由能曲线的变化。【拟解决的关键问题】应用PFC模型模拟取向角为4°的双晶对称的亚晶界在外加应力作用下亚晶界的运动以及湮没的过程,同时从能量变化角度分析其变化过程的规律。
1 模型与方法 1.1 体系的能量密度函数根据文献[18],原子密度场函相场的表达式可写为
| $ \rho = {\sum _{n, m}}{a_{n, m}}{e^{i\mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\rightharpoonup$}} \over G} \cdot \mathord{\buildrel{\lower3pt\hbox{$\scriptscriptstyle\rightharpoonup$}} \over r} }} + {\rho _0}, $ | (1) |
式子右边第一项是原子的周期排列特征项,第二项是反映均匀相的原子密度。体系的自由能函数F表示为
| $ F = \int {\left\{ {\frac{\rho }{2}\left[ {\gamma + {{\left( {1 + {\nabla ^2}} \right)}^2}} \right]\rho + \frac{{{\rho ^4}}}{4}} \right\}{\rm{d}}r, } $ | (2) |
其中γ代表温度的参数。
保守的原子密度场变量的演化可用与时间相关的Cahn-Hilliard动力学方程描述:
| $ \frac{{\partial \rho }}{{\partial t}} = {\nabla ^2}\frac{{\delta F}}{{\delta \rho }}。$ | (3) |
可以利用式(3)将动力学方程(4)写为
| $ \frac{{\partial \rho }}{{\partial t}} = {\nabla ^2}\left( {\gamma \rho + {{\left( {1 + {\nabla ^2}} \right)}^2} + {\rho ^3}} \right)。$ | (4) |
由于方程(4)是高阶的非线性偏微分方程,我们将采取半隐式伪谱法[18]来求解该动力学方程。在本研究中,我们将空间步长Δx,Δy均取为π/4,时间步长为0.5,模拟区域为1 024Δx×1 024Δy。六角相的单相原子密度函数形式为
| $ \begin{array}{l} \;\;\;\;\;\rho = A[{\rm{cos}}\left( {qx} \right){\rm{cos}}\left( {qy/\sqrt 3 } \right) - \\ {\rm{cos}}\left( {2qy/\sqrt 3 } \right)/2 + {\rho _0}], \end{array} $ |
其中,波矢q=
在外加应变的条件下,晶粒变形,引起晶界、亚晶界的位错运动。模拟中将采取等面积不变模型[5],即ΔxΔy=Δx'Δy',其中Δx,Δy是变形前的空间步长,Δx', Δy'是变形后的空间步长。设
|
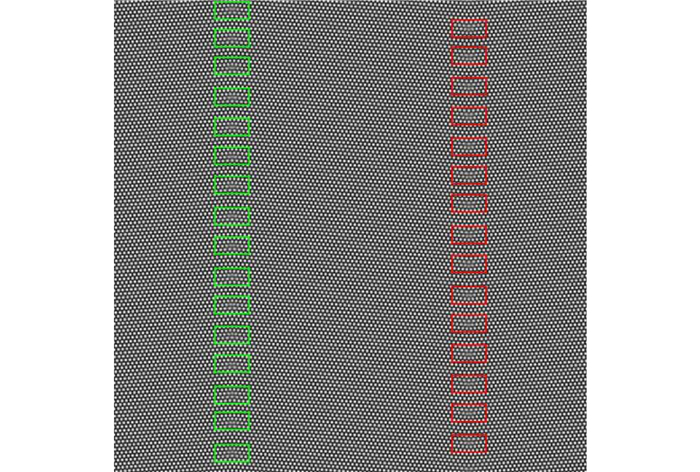
图 1 样品的晶界位错排列 Fig.1 The arrangement diagram of grain boundary dislocation of sample |

|
图 2 自由能-时间变化曲线 Fig.2 The curve of free energy-time change |

|
绿色方框和圆圈分别示意A组和C组位错,红色方框和圆圈分别示意B组和D组位错,白色箭头代表位错的攀移方向或者滑移方向
The green block and circle represent A group dislocations and C group dislocations respectively.The red block and circle represent B group dislocations and D group dislocations respectively.The white arrow represents the climbing direction or slip direction of the dislocation (a)ε=0;(b)ε==0.040 5;(c)ε=0.068 8;(d)ε=0.070 6;(e)ε=0.072 7;(f)ε=0.076 7;(g)ε=0.108 8; (h)ε=0.123 5;(i)ε=0.134 3;(j)ε=0.145 0 图 3 4°对称倾侧晶界位错随时间的演化过程 Fig.3 The evolution process of dislocations motion at 4° orientation angle |
在外加应力作用下,整个体系的畸变能不断增加[19](图 2中a~b段),总的自由能上升,当应力达到一定值时导致位错发生滑移(图 2中c点),使得畸变能降低(图 2中c~f段)。随着应力的不断增加,当施加的应力足以克服晶界的位错复分解的阻力时,左右两条晶界中各有一半的位错有序地从两条晶界发射,余下的位错则将继续沿着晶界攀移;从晶界发射的位错,继续滑移运动直至遇到伯格斯矢量大小相等方向相反的位错从而发生湮没。详细的晶界湮没过程如图 3a~j所示。
图 2中a~j点均与图 3a~j一一对应。由图 3可见,4°晶界的湮没过程可主要分为3个过程:1)晶界位错攀移(图 3a~c);2)位错分解,滑移,湮没(图 3c~f);3)剩下一半的晶界位错继续攀移,分解,滑移,湮没(如图 3f~j),最后形成单晶。在图 3a~b位错发生攀移,由于攀移会使整体的自由能升高[19],所以相对应的图 2中a~b段的自由能曲线在升高。当ε=0.040 5时(图 3c),位错分为4类,分别用绿色的矩形、圆形和红色的矩形、圆形表示出来。其中矩形所圈出来的位错就是即将发生分解的两组位错,将左侧的位错命名为A组,右侧的命名为B组。A组中即将分解的位错已经由两个位错(图 3c中细节图A1),变为4个位错(图 3c中细节图A2),B组也是同样的规律,这些位错增加的都是一对伯格斯矢量完全相反的位错,所以伯格斯矢量仍然守恒。其中A组位错将分解出A1、A2位错,B、C、D组也是同样的规律,这些分解出来的位错形成了亚晶界。在图 3c~d的过程中,分解出来的位错向两侧滑移,滑移的方向已经用白色的箭头示意。并且我们可以观察到,之前的样品具有两条晶界,随着应力的增加,部分位错进行滑移,产生了新的亚晶界,新增的两条绿色与两条红色虚线就是亚晶界的所在处,亚晶界也随着位错的滑移进行运动。晶体被分为7个部分,从左到右分别为晶界1~6,最后一个部分由于边界周期性所以还是晶界1。可见,晶体在实验过程中由双晶变为六晶,各个晶体的面积随着亚晶界的运动而变化。当应力为0.072 7时(图 3e),亚晶界随着分解的位错运动而移动,A2组和B1组位错都是向中间运动。由于A2组中红色的位错与B1组黄色位错,以及A2组和B1组的绿色位错都是伯格斯矢量且大小相等方向相反,所以刚好发生湮没。分解的位错进行滑移运动时,初始的两组位错(即绿色和红色圆圈圈出来的位错)正在进行攀移运动,左侧的位错向下攀移,右侧的向上攀移,攀移的方向如图中箭头所示。在图 3e~f过程中,位错进行了滑移和湮没,因此自由能一直处于下降的趋势。当中间只剩下3对即将湮没的位错时(图 3f),自由能也到达了谷底。所有的亚晶界即将完全消失。当应力为0.108 8时,样品只剩下图中圈出来的8对位错,在图 3f~g的过程中,8对位错进行着攀移运动,左边绿色圈出的位错向下攀移,右边红色圈出的位错向上攀移,将左侧位错命名为C组,右侧位错命名为D组。应力加到一定的程度后,位错再次发生分解(图 3h),将分解的位错分别命名为C1、C2、D1、D2。随着分解的位错运动,样品由两条晶界,变为4条亚晶界,各组位错滑移的方向由白色箭头标出。当应力为0.134 3时,在图 3h~i的过程中,位错一直进行着滑移运动,亚晶界也在运动,所以自由能也一直处于下降的趋势。C2和D1组位错组成的亚晶界向中间滑移运动,C2组的红色位错与D1组的黄色位错,以及C2组与D1组的绿色位错都是伯格斯矢量大小相等方向相反的位错,刚好可以完成湮没。如图 3j所示,位错已经全部湮没,形成单晶。
3 结论施加应力下的小角双晶晶界湮没过程,主要分为3个阶段特征:第一阶段是体系能量增加的阶段,反映了位错的攀移和滑移过程,以及生成的亚晶界迁移; 第二阶段是体系自由能量降低的阶段,反映了伯格斯矢量异号的亚晶界位错相互之间靠近吸引,发生湮没过程; 第三阶段是重复前两个阶段,最后位错全部湮没消失,形成完整单晶。
| [1] |
杨涛, 陈铮, 董卫平. 应力诱发双位错组亚晶界湮没的晶体相场模拟[J]. 金属学报, 2011, 47(10): 1301-1306. YANG T, CHEN Z, DONG W P. Phase field crystal simulation of stress-induced annihilation of sub-grain boundary with double-array dislocation[J]. Acta Metallurgica Sinica, 2011, 47(10): 1301-1306. |
| [2] |
高英俊, 周文权, 邓芊芊, 等. 晶体相场方法模拟高温应变作用的预熔化晶界的位错运动[J]. 金属学报, 2014, 50(7): 886-896. GAO Y J, ZHOU W Q, DENG Q Q, et al. Phase field crystal simulation of strain effects on dislocation movement of premelting grain boundaries at high temperature[J]. Acta Metallurgica Sinica, 2014, 50(7): 886-896. |
| [3] |
高英俊, 黄礼琳, 周文权, 等. 高温应变下的亚晶界湮没与位错旋转机制的晶体相场模拟[J]. 中国科学, 2015, 45(3): 306-321. GAO Y J, HUANG L L, ZHOU W Q, et al. Phase field crystal simulation of subgrain boundary annihilation and dislocation rotation mechanism under strain at high temperature[J]. Sci Sin Tech, 2015, 45(3): 306-321. |
| [4] |
LIU D H, WANG A G, YU J C, et al. Molecular dynamics simulation on formation mechanism of grain boundary steps in micro-cutting of polycrystalline copper[J]. Computational Materials Science, 2017, 126: 418-425. DOI:10.1016/j.commatsci.2016.10.001 |
| [5] |
LI J, FANG Q, LIU B, et al. The effects of pore and second-phase particle on the mechanical properties of machining copper matrix from molecular dynamic simulation[J]. Appl Surf Sci, 2016, 384: 419-431. DOI:10.1016/j.apsusc.2016.05.051 |
| [6] |
HU S, CHEN Z. Phase-field-crystal study on the reaction mechanisms of opposite sign edge dislocations appearing in the deformation processes of asymmetric tilt sub-grain boundary system[J]. Computational Materials Science, 2016, 124: 193-203. |
| [7] |
CHEN L Q. Phase-field models for microstructure evolution[J]. Annual Review of Materials Research, 2002, 32(1): 113-140. DOI:10.1146/annurev.matsci.32.112001.132041 |
| [8] |
HIROUCHI T, TAKAKI T, TOMITA Y. Developme- nt of numerical scheme for phase field crystal deformation simulation[J]. Computational Materials Science, 2009, 44(4): 1192-1197. DOI:10.1016/j.commatsci.2008.08.001 |
| [9] |
BERRY J, ELDER K R, GRANT M. Melting at dislocations and grain boundaries:A phase field crystal study[J]. Physical Review B, 2008, 77(22): 224114. DOI:10.1103/PhysRevB.77.224114 |
| [10] |
WU K A, ADLAND A, KARMA A. Phase field crystal model for fcc ordering[J]. Phys Rev, 2010, 81: 061601. DOI:10.1103/PhysRevA.81.061601 |
| [11] |
ELDER K R, HUANG Z F. A phase field crystal study of epitaxial island formation on nanomembranes[J]. Journal of Physics:Condensed Matter, 2010, 22(36): 364103. DOI:10.1088/0953-8984/22/36/364103 |
| [12] |
GAO Y J, LUO Z R, HUANG L L, et al. Phase field crystal study of nano-crack growth and branch in materials[J]. Modell Simul Mater Sci Eng, 2016, 24: 055010. DOI:10.1088/0965-0393/24/5/055010 |
| [13] |
高英俊, 袁龙乐, 刘瑶, 等. 不同温度晶界位错湮没过程的晶体相场模拟[J]. 广西科学, 2014, 21(3): 203-208. GAO Y J, YUAN L L, LIU Y, et al. Phase field crystal simulation of dislocation annihilation at different temperature[J]. Guangxi Sciences, 2014, 21(3): 203-208. |
| [14] |
刘瑶, 袁龙乐, 卢强华, 等. 晶体相场模拟取向角对晶界湮没过程的影响[J]. 广西科学, 2016, 23(5): 437-442. LIU Y, YUAN L L, LU Q H, et al. Phase field crystal simulation of effect of differrent orientation angle on annihilation of grain boundary[J]. Guangxi Sciences, 2016, 23(5): 437-442. |
| [15] |
杨瑞琳, 刘瑶, 胡绪志, 等. 双位错滑移运动的晶体相场模拟[J]. 广西科学, 2016, 23(5): 443-447. YANG R L, LIU Y, HU X Z, et al. Phase-field-crystal simulation of double dislocation Gliding[J]. Guangxi Sciences, 2016, 23(5): 443-447. |
| [16] |
HU S, XI W, CHEN Z, et al. Coupled motion of grain boundaries and the influence of microcracks:A phase-field-crystal study[J]. Computational Materials Science, 2017, 132: 125. DOI:10.1016/j.commatsci.2017.02.021 |
| [17] |
黄礼琳, 叶里, 胡绪志, 等. 不同晶向取向的裂纹扩展演化模拟[J]. 广西科学, 2016, 23(5): 454-458. HUANG L L, YE L, HU X Z, et al. Simulation study of crack propagation and evolution in different crystal orientations[J]. Guangxi Sciences, 2016, 23(5): 454-458, 469. |
| [18] |
任秀, 王锦程, 杨玉娟, 等. 纯物质晶界结构及运动的晶体相场法模拟[J]. 物理学报, 2010, 59(5): 3595-3600. REN X, WANG J C, YANG Y J, et al. Simulation of the structure and motion of grain boundary in pure substances by phase field crystal model[J]. Acta Physica Sinica, 2010, 59(5): 3595-3600. |
| [19] |
孔令一, 卢昱江, 邓芊芊, 等. 二维大取向角对晶界湮没过程的晶体相场模拟[J]. 广西科学院学报, 2017, 33(4): 228-233. KONG L Y, LU Y J, DENG Q Q, et al. Phase field crystal simulation of grain boundary annihilation with two dimensional large orientation angle[J]. Journal of Guangxi Academy of Science, 2017, 33(4): 228-233. |
 2018, Vol. 34
2018, Vol. 34 


